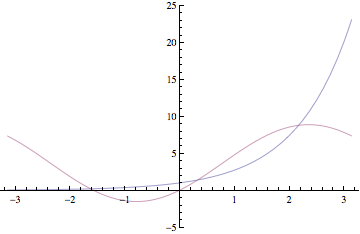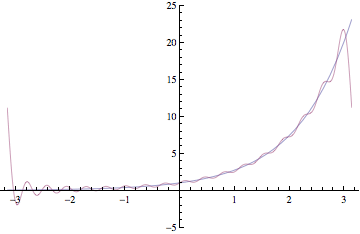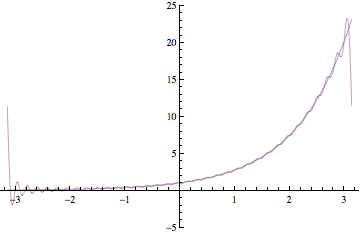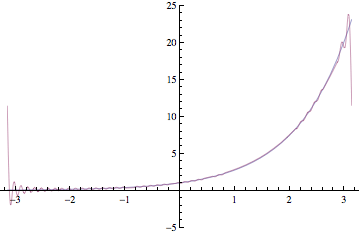


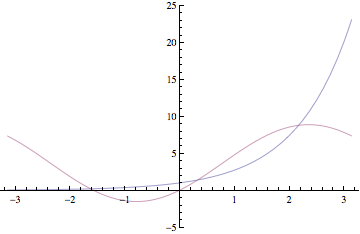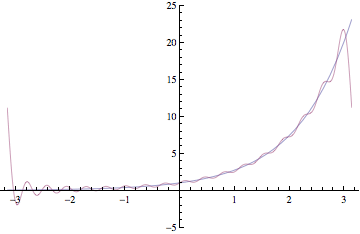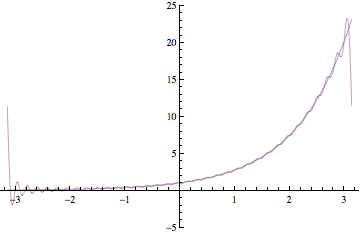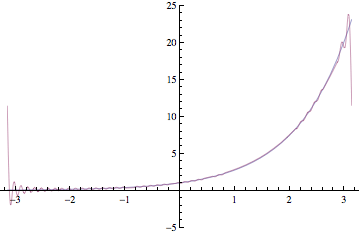
| Section | Problems to do | Target date | Comments |
|---|---|---|---|
| 1.1 | 1,2,3,4,5,6,7,9 | Tuesday, August 31 | |
| Gaussians | 1,2 | Thursday, Sept 2 | |
| Flux | 1,2,3 | Thursday, Sept 9 | |
| 1.2 | 1,3,4,6 | Friday, Sept 10 | |
| 1.2 | 5,7,8 | Tuesday, Sept 14 | |
| 1.3 | 3,4,5,6,7 | Monday, Sept 20 | |
| 1.5 | 3,4,5 | Monday, Sept 27 | |
| 1.9 | 1,2,3,5 | Thursday, Sept 30 | |
| 2.1 | 1,2,3,4,5 | Monday, October 11 | |
| 2.4 | 1,2,4 | Thursday, October 21 | |
| 2.2 | 2,3,5 | Thursday, October 21 | For Problem 2, explicitly evaluate the integral in | (2.14) for the given initial velocity (rather than relying on numerical approximation). |
| 3.2 | 1,4,5,8 | Monday, November 1 | |
| 3.3 | 1,2,5 | Friday, November 5 | |
| 3.4 | 3,6,7,9 | Monday, November 15 | |
| 4.1 | 1,2,3 | Monday, November 22 | |
| 4.2 | 1,2,4 | Monday, November 22 | |
| 4.3 | 1,2,3,4 | Thursday, December 2 | |
| 4.4 | 1,2,3 | Friday, December 3 |
We finished the course by finding normal modes for vibrations of a circular membrane in the general case. This involves analyzing a PDE for an unknown function of three variables. In a product solution approach, we need to do separation of variables in two stages so we have two separation constants and two SL problems. The eigenvalues from one of these feed into the other and then the eigenvalues from the second feed into the remaining T-problem.
The annual end-of-semester Math/CS department gathering is Wednesday at 5 pm in TH 390. Come have some food and conversation. (Who knows, there may even be ice cream.)
Exam #11 is due on Monday, December 13. Note that this is the end of the final exam period scheduled for this course. Reasonable requests for extensions will be considered. Also, note that you again have a choice of two problems for this exam.
Here are office hours for the remainder of the week:
| Wednesday December 8 | 2:30-3:30 pm |
| Thursday December 9 | 2:30-4:00 pm |
| Friday December 10 | 10:30-11:30 am and 2:30-3:30 pm |
I will have other times that I can be available by appointment. Call or email to set up a time.
Today, we finished up our analysis of rotationally symmetric vibrations of a circular membrane. Our focus was on finding and understanding normal mode solutions. These correspond to the individual products solutions. We could also use a combination of product solutions to satisfy given initial conditions. Tomorrow, we will look at the general case in which solutions do not necessarily have rotational symmetry.
Exam #11 is due on Monday, December 13. Note that this is the end of the final exam period scheduled for this course. Reasonable requests for extensions will be considered. Also, note that you again have a choice of two problems for this exam.
In class, we began analyzing the problem of vibrations on a circular membrane. These are modeled with the wave equation in two spatial variables. To simplify things, we will first look for solutions that have rotational symmetry around a vertical axis through the center of the circular membrane.
After setting up an IBVP in polar coordinates, we set in to finding solution using our familiar process. We soon encountered a new feature in the SLP problem, namely a linear, homogeneous, non-constant coefficient second-order ODE that is not easy to solve. The conventional approach to solving this ODE starts by looking for solutions in the form of a power series. Some detailed analysis (that we will not carry out) reveals two independent solutions in the form of power series. (Well, one of them is a power series while the other includes negative powers so technically not a power series.) The two solutions are called Bessel's function of the first kind of order 0 and Bessel's function of the second kind of order 0. Properties of these functions are well established. We used Mathematica to get some insights on them.
Bessel functions are introduced in Section 4.5 as part of analyzing the heat equation on a disk.
Exam #10 is due on Monday, December 6. Note that you have a choice of two problems for this exam.
Today, we first fixed a mistake in Monday's transformation of Laplace's equation to polar form. With the correct form in hand, we finished our solution of Laplace's equation on a disk.
Exam #10 is due on Monday, December 6. Note that you have a choice of two problems for this exam.
Today, we continued with analyzing Laplace's equation on a disk but did not get too far because we had to pause for instructor evaluations.
Exam #10 is due on Monday, December 6. Note that you have a choice of two problems for this exam.
In class, we started analyzing Laplace's equation on a disk. Since polar coordinates provide a convenient way of describing a disk, we first converted Laplace's equation from our familiar cartesian expression to the equivalent expression in polar coordinates. The main tool we used is the chain rule. With the polar form of Laplace's equation in hand, we then formulated a complete BVP. The boundary conditions include one condition for the physical boundary at the edge of the disk and three other conditions corresponding to non-physical "coordinate boundaries".
After formulating a complete BVP, we began looking for a solution using a process that should be familiar by now. We'll continue with this tomorrow.
Exam #10 is due on Monday, December 6. Note that you have a choice of two problems for this exam.
After break, we'll pick up with a BVP for Laplace's equation on a disk.
Exam #10 is due on Monday, December 6. Note that you have a choice of two problems for this exam.
In class, we had two homework solution presentations and then we worked through the details of Section 4.2#2
In class, we finished solving a BVP for Laplace's equation on a rectangular region. We then interpreted and visualized our solution.
Exam #9 is due on Friday, November 19. Note that this exam involves a bit more than recent exams.
We started class by using the D'Alembert form to visualize a specific solution of our wave equation IBVP. You then started in on solving a BVP for Laplace's equation using the ideas we've been developing over the past week. We'll finish this up tomorrow.
Exam #9 is due on Friday, November 19. Note that this exam involves a bit more than recent exams.
Today, we returned to our solution of an IBVP for the wave equation to get some interpretation. We first looked at the individual product solutions. In the context of the wave equation, these are often called normal modes. Each normal mode is a standing wave vibrating at a specific frequency. The time-dependent factor can be understood as a linear combination of cosine and sine terms or as a shifted cosine.
Toward the end of class, we used trigonometric identities to rewrite our full specific solution in the D'Alembert form consisting of right- and left-moving wave trains. We'll visualize this more carefully on Thursday.
Exam #9 is due on Friday, November 19. Note that this exam involves a bit more than recent exams.
In class, we first looked at the details of Section 3.4 #6. Today's Mathematica file shows some of our results. We then turned to solving an IBVP for the wave equation using the same approach we've been applying to IBVP for the heat equation. We'll spend some time tomorrow analyzing our solutions.
Exam #9 is due on Friday, November 19. Note that this exam involves a bit more than recent exams.
In class, we proved a small piece of the SL theorem and then you worked with this example of a regular SL problem.
Over the weekend, I'll post a handout with the version of the SL theorem that we have discussed in class.
Exam #9 is due on Friday, November 19. Note that this exam involves a bit more than recent exams.
We started class by returning to the X-problem that arose in solving our first example of an IBVP for the heat equation. Without explicitly solving this problem, we were able to prove that orthogonality for any pair of eigenfunctions corresponding to distinct eigenvalues. We were also able to prove that the separation constant must be negative to get nontrivial solutions. This example illustrates some of the ideas of Sturm-Liouville theory.
We then wrote down a version of the Sturm-Liouville theorem for regular Sturm-Liouville problem. The theorem tells us about the existence and nature of eigenvalues and eigenfunctions that come out of a SL problem. As a practical tool, we can use the SL theorem to guarantee orthogonality and maximality of sets of eigenfunctions without having to do an explicit inner product calculation.
We began class by redoing part of the analysis from our first IBVP example using a linear algebra approach. In this approach, we get eigenvalues from a condition that the determinant of a certain coefficient matrix is zero (in order to get nontrivial solutions for a homogeneous system of equations).
You then worked out the details of a second IBVP for the heat equation. This lead us to a new set of functions \(\{\sin(\frac{(2n-1)\pi}{2l}x)\bigl|n=1,2,3,\ldots\}\). Although we did not check explicitly, this set turns out to be orthogonal in L2[0,l]. The Sturm-Liouville theorem that we discuss on Thursday will provide us a guarantee of this orthogonality without having to do an inner product calculation to check orthogonality explicitly.
Today, we finished off the IBVP for the heat equation that we started yesterday. This example sets a pattern that we will follow in other problems. Part of this pattern is finding an orthogonal set of functions by solving a BVP for a second-order ODE. (In our first example, this was the X-problem.) Later this week, we'll discuss some general theory behind this part of the process.
Today, we first reviewed the Gram-Schmidt process from linear algebra. In the G-S process, we start with a linearly independent set (in some vector space) and then compute an orthogonal set (using an inner product for the vector space). This is one way of generating orthogonal sets. You'll get to do an example of this in Exam #8.
We next turned our attention to solving an IBVP for the heat equation on a bounded interval. We started by looking for solutions having the product form u(x,t)=X(x)T(t). We ran this form through the PDE and the boundary conditions to get conditions that X(x) must satisfy. These conditions consist of a second-order linear ODE along with two boundary conditions. The ODE includes a constant λ that came in through the separation of variables argument. By directly analyzing the ODE and BCs, we determined that the BVP has non-trivial solutions only for certain values of λ. We will call these the eigenvalues of the BVP with the corresponding solutions called eigenfunctions. On Monday, we'll finish off this example by analyzing the conditions on T(t) and putting everything together to satisfy the initial condition.
In class, we looked at the special case of Fourier series as the historical first examples studied of orthogonal expansions in L2. Fourier did his work before the general context of vector spaces and inner products was developed. One point of our discussion was a pointwise convergence result for a Fourier series from a function defined on an interval [-l,l]. We also looked at pointwise convergence of the Fourier sine series and the Fourier cosine series for a function defined on an interval [0,l]
Today, we did two calculations that lead to a particular conclusion: Among all possible ways of choosing coefficients in a partial sum of orthogonal functions, the choice that minimizes mean-square error is the coefficients calculated as ak=⟨ f , fk ⟩/⟨ fk , fk ⟩
In class, we explored issues of convergence for orthogonal expansions in L2. The context is that we start with function f and then compute the coefficients ak so that we can construct the orthogonal expansion OE, which is a function itself. By looking at examples, we saw that an orthogonal expansion OE does not necessarily converge to the function f for all x in the relevant domain. In other words, the orthogonal expansion does not necessarily converge pointwise to f. We then began looking at a different notion of convergence in which the difference between OE(x) and f(x) is measured using the norm given by our L2 inner product. This type of convergence is called normwise convergence in a general context. In the specific context of our L2 inner product, it can also be called mean-square convergence (because the distance in this case is essentially averaging the square of the difference between two functions).
We did not have class today to give those who are interested the opportunity to attend 2010 Race & Pedagogy National Conference events that will be taking place on campus.
Today, you computed the othogonal expansion of a piecwise constant function in terms of the set {sin(kx)}∪{cos(kx)}∪{1}. On Monday, we'll look at the relationship between the original function f and its orthogonal expansion.
I've assigned some problems from Section 3.1. Bring questions from these to class on Monday.
Here is Exam #7. It is due on Thursday, November 4.
We will not have class tomorrow to give those who are interested the opportunity to attend 2010 Race & Pedagogy National Conference events that will be taking place on campus.
In class, we showed that the set {sin(kx)} in L2(-π,π) is orthogonal with respect to the standard inner product. We then looked at two examples of expanding a function in terms of this orthogonal set. The first was the function you experimented with using Mathematica last week. This seemed to work nicely in the sense that we as we add more terms, partial sums for the expansion series become better approximations for the function. In a second example, we tried expanding cos(x) in terms of {sin(kx)}. This fails miserably because all of the expansion coefficients evaluate to 0. In other words, cos(x) is orthogonal to every element in {sin(kx)}. To deal with this, we added more things to form a larger orthogonal set, namely {sin(kx)}∪{cos(kx)}∪{1}. This turns out to be a maximal (or complete) orthogonal set in L2(-π,π).
We next need to turn attention to convergence issues since our orthogonal expansions in L2 are infinite series. We'll look at this tomorrow.
We will not have class on Friday, October 29 to give those who are interested the opportunity to attend 2010 Race & Pedagogy National Conference events that will be taking place on campus.
Today, we first looked at an example of orthogonal expansion in R3 using the dot product. We then reviewed some general ideas from linear algebra: vector space, inner product, and orthogonal basis. With an orthogonal basis, we can expand any other element in the vector space by using the inner product to compute the expansion coefficients. This is much more efficient than solving a system of equations for the coefficients, which is the approach to take with a non-orthogonal basis. Lastly, we began exploring the vector space L2 equipped with the inner product that is a natural generalization of the dot product. This handout has a few notes on the vector space L2.
Here is Exam #6 in case you did not get the email last Friday.
We will not have class on Friday, October 29 to give those who are interested the opportunity to attend 2010 Race & Pedagogy National Conference events that will be taking place on campus.
We need to develop some new mathematical tools. As an introduction to this, we went to the computer lab where you did some work in Mathematica to experiment with approximating a given function using a linear combination of sine functions. As homework, you should finish up this experimentation (from the handout) and bring your coefficient values to class on Monday.
We will not have class on Friday, October 29 to give those who are interested the opportunity to attend 2010 Race & Pedagogy National Conference events that will be taking place on campus.
After looking at questions from homework problems, we turned our attention to an IBVP for the heat equation on a bounded interval. Our first thought was to relate this new problem to an old problem, namely the Cauchy problem for the heat equation. So, we began thinking about how to extend the intial condition for the given interval 0 < x < 0 to an initial condition for all x in such a way that the given boundary conditions would be satisfied by the evolving solution. This seemed to be a complicated task so we abandoned ship. Instead, we will develop a new approach. Part of this new approach will require us to develop some new mathematical tools. We'll start in on this tomorrow.
We are going to skip over the rest of Chapter 2 for now. If time permits, we'll circle back toward the end of the semester to talk about integral transform approaches to solving PDEs on unbounded domains.
Today, you worked out the specific solution for the general Cauchy problem for the wave equation (in one spatial dimension). We use characteristic coordinates to get the general solution and then apply the given initial conditions. The specific solution has two pieces which can be interpreted as
Today, we solved the general IBVP for the heat equation on the half-line with boundary condition u(0,t)=0 for t > 0. We did this building the odd extension of the initial condition so we have a new initial condition defined for all x. This gives us a Cauchy problem for which we already have the solution. Using the odd extension insures that the boundary condition is satisfied.
We've skipped ahead to Section 2.4 in the text. We'll soon come back to the ideas in Sections 2.2 and 2.3.
In class, we went a bit more slowly through the last step of the process that took most of last week: Make one last (big) generalization to heat energy spread out over a continuum of points so the initial condition is u(x,0)=u0(x) and the solution is \[ u(x,t)=\int_{-\infty}^\infty u_0(y)G(x-y,t)\,dy. \] We can read this as saying that the solution is a continuum superposition of evolving Gaussians shifted by y and scaled by u0(y)dy. This is a powerful result that can take some time and experience to become comfortable with. The problems from Section 2.1 will give you some of that experience.
In class, we went a bit more slowly through the last step of the process that took most of last week: Make one last (big) generalization to heat energy spread out over a continuum of points so the initial condition is u(x,0)=u0(x) and the solution is \[ u(x,t)=\int_{-\infty}^\infty u_0(y)G(x-y,t)\,dy. \] We can read this as saying that the solution is a continuum superposition of evolving Gaussians shifted by y and scaled by u0(y)dy. This is a powerful result that can take some time and experience to become comfortable with. The problems from Section 2.1 will give you some of that experience.
In class, we derived the solution for a general heat equation Cauchy problem. The full derivation has taken several days. Here's an outline of the steps:
At the end of class today, we made a quick argument for this last step. On Monday, we'll go through that argument again with a bit more detail.
We began class by finishing off the heat equation Cauchy problem that we started on Tuesday. One hitch is that our general solution from Tuesday is not defined for t=0. So, we did the next best thing: we set the limit of the general solution as t→0 equal to the given initial condition and used this to determine specific values for the constants that appear in the general solution.
Toward the end of class, we generated a second heat equation solution by differentiating our first solution. This second solution should be familiar from Problem 1 of Section 1.1. Tomorrow, we will try to make more sense of the initial condition that this solution satisfies (again, in the limit as t→0).
I've assigned one problem from Section 2.1. I'll assign more after class tomorrow.
We have gotten a small taste of dimensional analysis with focus on finding dimensionless quantities. Our main use of this tool will be in coming up with an ansatz to solve a specific Cauchy problem for the heat equation. We started in on this today and came up with a general solution to the heat equation. Tomorrow, we will apply the initial condition to determine specific values for the constants in our general solution.
Today, we stepped through Friday's dimensional analysis example with a more systematic approach and we then started in on a more involved example. For homework, you should finish this example by
For reference, here is the relevant coefficient matrix and its row reduced form:
\[ \left( \begin{array}{ccccccc} 1 & 0 & 0 & 0 & 0 & 1 & 1 \\ 1 & 3 & 0 & 0 & 3 & 7 & 4 \\ -2 & 0 & 0 & 0 & 0 & -2 & -2 \\ 0 & 0 & 1 & 0 & 0 & 0 & -1 \\ 0 & 0 & 0 & 1 & -1 & -2 & -1 \end{array} \right) \textrm{ row reduces to } \left( \begin{array}{ccccccc} 1 & 0 & 0 & 0 & 0 & 1 & 1 \\ 0 & 1 & 0 & 0 & 1 & 2 & 1 \\ 0 & 0 & 1 & 0 & 0 & 0 & -1 \\ 0 & 0 & 0 & 1 & -1 & -2 & -1 \\ 0 & 0 & 0 & 0 & 0 & 0 & 0 \end{array} \right) \]When I have time, I will post a handout with some of the details we went through in class to arrive at this coefficient matrix. For now, rely on your notes to remind yourself about how the dimensions (mass, length,...) correspond to the rows and the quantities (pressure, volume, ...) correspond to the columns.
You now have Exam #4. It is due on Thursday, October 7.
Our next major goal for the course is to analyze the Cauchy problem for the heat equation in one spatial variable. The Cauchy problem consists of the heat equation for -∞<x<∞, t>0 together with an initial condition u(x,0)=u0(x) for -∞<x<∞. This is a pure initial value problem since the unbounded domain in x requires no boundary conditions.
Our approach to analyzing the Cauchy problem for the heat equation will start with a sidenote on dimensional analysis. We saw one quick example today and will go through one or two others a bit more systematically on Monday.
You now have Exam #4. It is due on Thursday, October 7.
The Section 1.9 problems give you practice in classifying a given second-order linear PDE (as hyperbolic, parabolic, or elliptic) and in finding characterisitic coordinates that transform the second-order terms of the PDE to a standard form. One of the big lessons in all of this is that it is enough to understand how to analyze a prototype for each of the categories since other equations can be related to one of the prototypes. So, for the remainer of the course, we will focus on the prototypes:
Focussing on these three is much less limited than it might initially appear.
I'll distribute Exam #4 tomorrow.
Bring questions from Section 1.9 problems to class on Thursday.
After a quick review of what we did Friday on classifying quadratic curves, we turned attention to classifying second-order linear PDEs. We used the same tools for this new problem, although we worked in a more "nuts and bolts" way that is not quite as elegant as the process we outlined for quadratic curves. (The quadratic curve classification process would require "nuts and bolts" if we want to find the eigenvectors of the quadratic form Q in order to give the full geometry of the curve (e.g. principal axes in the case of an ellipse).
In class, you finished the somewhat messy calculation that puts us in position to wisely choose characteristic coordinates. You should read Section 1.9 and work on the assigned problems to master the details of how to make good choices. The text lays out a good choice for each of the three main categories of second-order linear PDEs: elliptic, hyperbolic, and parabolic.
I got a bit behind in adding links for Mathematica files we create or view in class. I've now gone back and added previously missing links. Remember that you will need to open these files using Mathematica for them to be of use.
We started class by completing the characteristic coordinates analysis of the wave equation. We then turned attention to characteristic coordinates for more general second-order linear PDEs. The mathematical analysis of this is essentially the same as that used in classifying quadratic curves. In class, we used some nice linear algebra to get a handle on classifying quadratic curves as ellipses, parabolas, or hyperbolas. This handout has more details. (The last part of the handout deals with recognizing the shape of curves that are given parametrically by solutions to linear systems of two ODEs. This is not relevant to what we are doing.)
In class, we looked at modeling vibrations on a string under tension as a way of getting to the wave equation. We then started in on analyzing the wave equation using characteristic coordinates. We will finish this tomorrow and then turn attention to characteristic coordinates for general second-order linear PDEs. This will lead us to a classification of second-order linear PDEs into categories of hyperbolic, parabolic, and elliptic. This classification involves essentially the same mathematical analysis as the problem of classifying quadratic curves.
We've skipped a few things in Chapter 1 and done others out of order. Here's a quick guide to what we will and will not cover from the last sections of Chapter 1:
Our entry point for thinking about Laplace's equation is steady-state temperature distributions in two dimensions (using cartesian coordinates). In class, I briefly outlined some details on heat flow in two or more dimensions to give some sense of where Laplace's equation comes from. We will not be covering this in depth. If you are interested, there are more details in Section 1.7. Those of you who have taken, are taking, or will be taking advanced physics course might particularly benefit from reading this section.
The Section 1.8 problems on Laplace's equation deal with ideas we have not yet discussed. For now, try the following: Show that the function \[ u(x,y)= \frac{100}{\sinh(\pi\frac{W}{L})}\sin({\textstyle\frac{\pi}{L}}x) \sinh({\textstyle\frac{\pi}{L}}y) \] almost satisfies the Laplace equation boundary value problem that you set up and thought about in class today. To be specific, show that this function satisfies Laplace's equation for the rectangle 0<x<L, 0<y<W and that it satisfies three out of the four boundary conditions. (Note: Some groups used K rather than W.)
You have signed up for an individual meeting to work through the details of Exam #2. In light of this new task, I am moving the due date for Exam #3 to Friday. I will also delay beginning the first round of homework solution presentations until next week.
We spent the hour working through several problems from Section 1.3. Among the lessons of the day is the idea of using the basis {cosh(ax), sinh(ax)} as an alternative to the basis {e-ax,eax} for expressing the general solution of a particular second-order ODE. We'll find hyperbolic trig function basis to be useful in a number of places so you might want to review some basic facts about the hyperbolic trig functions.
In terms of the overall flow of the course, we are currently seeing how the three major second-order linear PDEs (heat/diffusion equation, Laplace's equation, wave equation) arise in modeling certain physical phenomena so that we can have some physical interpretation of problems we will be analyzing. Later this week, we'll see that these three equations are fundamental because any other second-order linear PDE can be transformed to one of these three with an appropriate change of coordinates. We'll soon finish this set-up of the main equations and then move on to the actual analysis of second-order PDE problems.
We now have an ordering for the first round of homework solution presentations. This weekend, I'll finish putting together the first few problems and will coordinate with the first few presenters on presentation dates. I'll also post a handout with comments on general expectations.
At the end of class, we quickly moved through a generalization of the heat equation to more than one spatial variable. To do this in a general way requires using some ideas from vector analysis that you may have only seen briefly at the end of multivariate calculus. Specifically, we need to use divergence and gradient. (If we want go back a step further to derive the fundamental conservation law for more than one spatial variable, we would also need to recall the divergence theorem (a.k.a Gauss's theorem in the physics world). At the end of the set=up, we used cartesian coordinates to get an expression that should seem like a natural generalization. Specifically, the expression uxx in the heat equation for one spatial dimension generalizes to uxx+uyy in the heat equation for two spatial variables. In this more general setting, we have u(x,y,t) generalizing u(x,t).
Bring questions on Section 1.3 problems to class on Monday.
In looking at Section 1.2 #8, we set up a model (PDE plus auxillary conditions), developed some intuition for the solution, and then started in on finding a solution explicitly. You should try to finish off that explicit solution and then compare with intuition.
Thinking about the diffusion equation in the context of heat energy flow provides a nice setting for interpreting various boundary conditions. In the example we set up today, we used a boundary condition for each end of a finite-length rod that prescribes the temparature at that end. Tomorrow, we'll look at other boundary conditions that arise naturally in setting up heat energy flow models.
Tomorrow, we'll start class with organizing the first round of homework solution presentations.
After addressing questions on problems from Section 1.2, we developed a simple relationship between flux and density as a model for diffusion. The relationship is that flux is proportional to the opposite of the rate of change in density (with respect to position). Using this relationship in the fundamental conservation law gives us the diffusion equation.
You now have Exam #2 in hand. The due date listed on that is changed to Friday, September 17.
In class, we used Mathematica to visualize solutions to Section 1.2 Problem 6. I'll post that Mathematica file after I've had time to add a few comments to it.
We then (finally) got to look at characteristic coordinates for a class of PDEs more general than our first example. I've assigned a few more problems from Section 1.2 that you should work on over the next few days.
You now have Exam #2 in hand. The due date listed on that is changed to Friday, September 17.
We spent a good portion of the class session working through the related problems of #3 and #6 in Section 1.2. The end result is a solution that takes some work to understand. You should work to better your understanding of the solution (and of the process we used to get it). As part of this, you might find it helpful to think about specific choices of the function g(t) that shows up in one of the auxillary conditions. For example, you could use the example g(t) is constant that we thought about in class. Another example would be to let g(t) equal 1 for t from 0 up to some value and then equal to 0 for all t greater than that value. In class on Monday, we'll briefly look at using computing technology to help in visualing a solution.
We did not have time to finish our more general look at characteristic coordinates. We'll do so on Monday. For now, keep pushing at the problems from Section 1.2.
In class, we looked at how to connect the fundamental conservation law to some physical intuition for the meaning of density and flux. We then looked at the fundamental conservation law in the case of advection and began developing characteristic coordinates as a tool for solving PDEs such as the advection equation.
I've assigned a few probems from Section 1.2. We'll start class tomorrow by addressing questions you bring on these problems. We'll then continue discussing characteristic coordinates.
Today, we derived the fundamental conservation law. This involves some basic accounted that relates total rate of change to the sum of two contributions, one accounting for change by flow and the other accounting for change by some (generic) creation/destruction process. With some mathematical moves, we can go from an integral version to a differential version so that we can understand the relevant relations on a point-by-point basis. What we discussed in class is covered Section 1.2 of the text (in somewhat briefer fashion).
To better understand the fundamental conservation law, you'll want to develop good intuition for density and flux. Your current assignment is problems from a handout on flux. To get a better feel for density, you might also find it helpful to look at this Math 181 handout on density and this Math 181 handout on computing total from density for non-uniform density. The problems on the first of these Math 181 handouts are trivial. The ones on the second handout involve some thinking and computing.
You had opportunity to get your hands on Mathematica today. In class, I'll continue to use Mathematica (and, occasionally, Sage) as a computational and visualization tool. You will find it very helpful to get basic mastery of some computing technology with equivalent capabilities.
We started class with a brief look at Sage. Sage is a freely available, open source mathematics software system. You can test out using Sage by setting up a (free) account to use a public Sage notebook server at sagenb.org For more details or to download a copy of Sage for you own machine, go to sagemath.org
Tomorrow, we'll start in the classroom to go over some basic ideas about using Mathematica and then go the TH 420 computer lab so you can try things for yourself. You should now have log-on privileges for the computers in TH 420 and card access to the room itself.
You should be working to finish up assigned problems from Section 1.1. Come talk with me if you have questions on any of these.
Whenever we look at Mathematica work in class, I'll post a copy of the notebook we generate. To make use of these, you'll need access to a working copy of Mathematica. (On Thursday, we'll talk about where you can find access on campus.) To use the file, you'll need to download it onto your local computer. Opening the file in your web browswer will give you something that is generally not human-readable.
For class on Thursday, you should continue to work on the Section 1.1 problems and you should work on the Gaussian function problems from the handout.
I am experimenting with a way of producing nicely typeset mathematics on web pages using a system called MathJax. If you are willing to be a guinea pig in this experiment, go to this sample page and then let me know what happens in your browser. Do you see a page of nicely typeset mathematics (after a few seconds of processing)? Something else?
I've assigned problems from Section 1.1. Start by reading the section to pick up some of the language used in the problem statements. Most of these problems are computational. Bring questions from the reading and problems to class on Tuesday. We can devote some, most, or all of Tuesday's class session to addressing questions, depending on how many are asked.
The Mathematical Atlas describes the many fields and subfields of mathematics. The site has numerous links to other interesting and useful sites about mathematics.
If you are interested in the history of mathematics, a good place to start is the History of Mathematics page at the University of St. Andrews, Scotland.
Check out the Astronomy Picture of the Day.